Ru About Tutor Works Blog
Readable

Surface intersection

I've made this article out of my twitter thread https://twitter.com/i_dianov/status/1367136469853351944

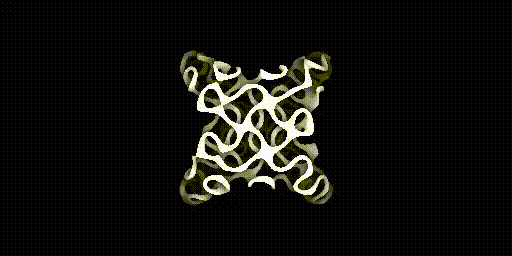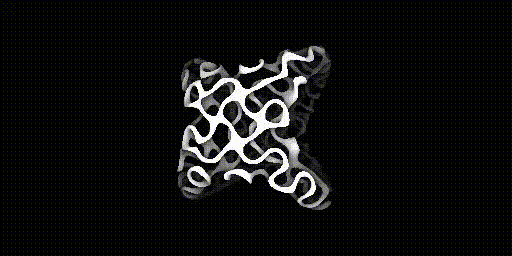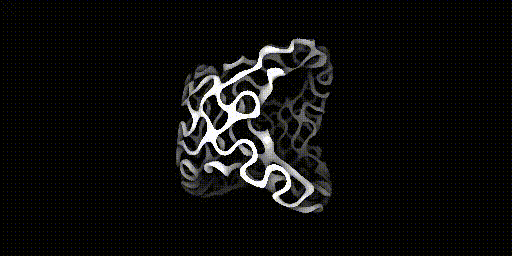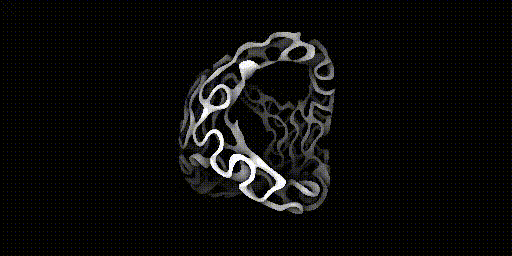
Intersection of sphere with intersaction of gyroid and sphere

Hey! This is a tutorial thread on how to find the intersection of two or more surfaces (not volumes) to make complicated #SDF shapes you can use for #raymarching.

  1. Let's start with a basic raymarching setup
#define SPHERE length(p)-1.
#define SDF(p) SPHERE
vec3 p; float d=0.,i,e=1.; for(;i++<99.&&e>.01; p=d*normalize(vec3((FC.xy-.5*r)/r.y,1))+ vec3(0,0,-5), p*=rotate3D(t,vec3(.3,1,0)), d+=e=.5*SDF(p)); o+=100./i/i;

https://bit.ly/3b9u7AL

You don't need to understand the bottom line, let's just focus on the line starting with

#define SDF(p) …

It defines the shape to render. Here's a sphere:

#define SPHERE length(p)-1.
#define SDF(p) SPHERE

  1. And here's an SDF for a plane Z (it's parallel to X- and Y-axis) https://bit.ly/3e4nd1D
#define PLANE_Z -p.z
#define SDF(p) PLANE_Z

  1. To find the intersection of these two surfaces we just do
#define SDF(p) length(vec2(PLANE_Z, SPHERE))

And BOOM!💥 The circle is here! https://bit.ly/3bXGTBJ

The thing is that length of two SDFs is close to zero only if both SDFs are close to zero.

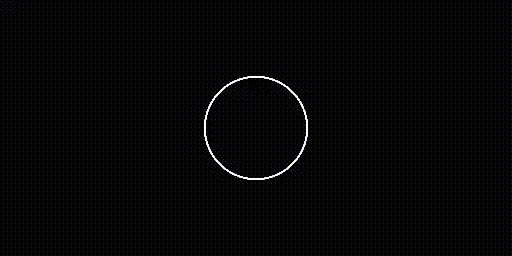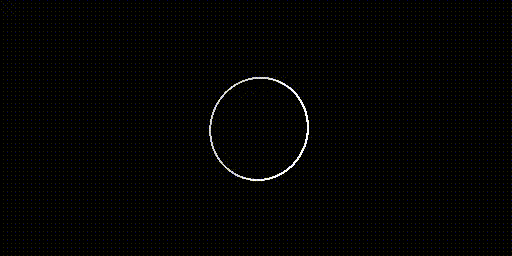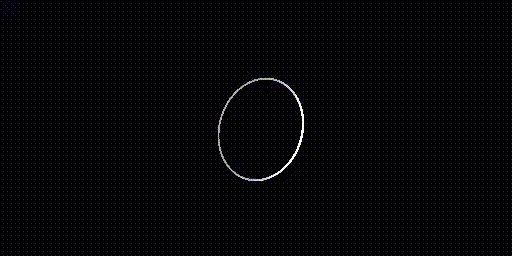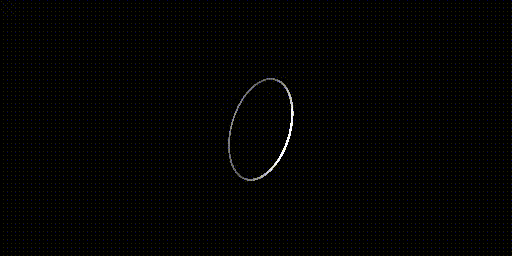
  1. We can inflate this surface by subtracting a small value from the SDF:
#define SDF(p) length(vec2(PLANE_Z, SPHERE))-.2

Please, pay attention to terminal -.2 in the formula. https://bit.ly/3bXGTBJ

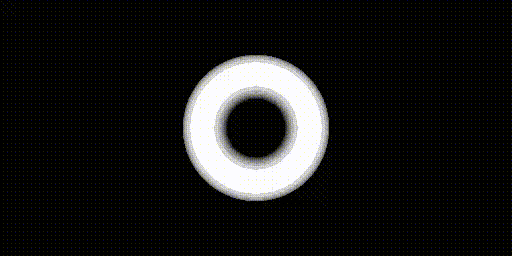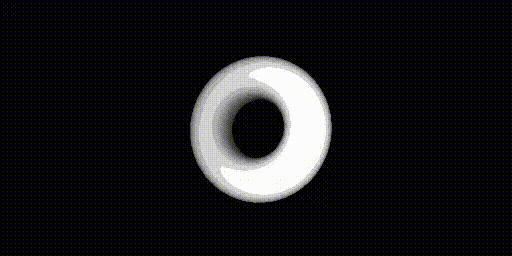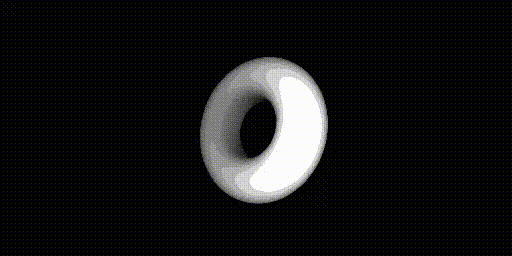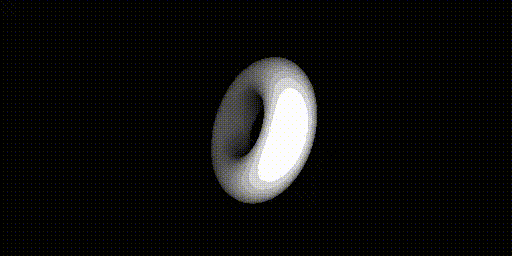
  1. Can you make a short break to think about how to make a cylinder using this trick?
  1. Great, you did it! It's just an intersection of two planes inflated by subtracting a small value:
#define SDF(p) length(vec2(PLANE_X, PLANE_Z))-1.2

They often shorten this to just length(p.xz)-1.2 which is effectively the same.

https://bit.ly/30me4cZ

  1. Let's intersect cylinders! It will result in two crossing circles
#define CYLINDER_Y length(vec2(PLANE_X, PLANE_Z))-1.2
#define CYLINDER_X length(vec2(PLANE_Y, PLANE_Z))-1.2
SPHERE))-.01
#define SDF(p) length(vec2(CYLINDER_Y, CYLINDER_X))-.1

https://bit.ly/3883WbT

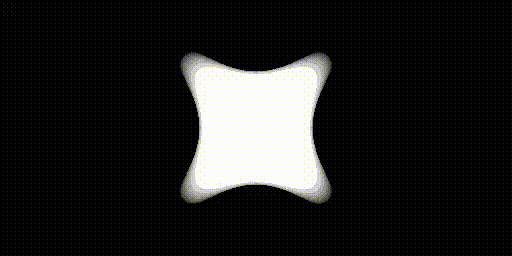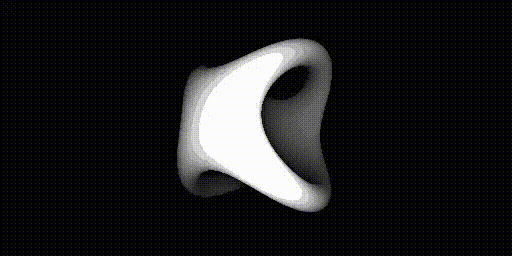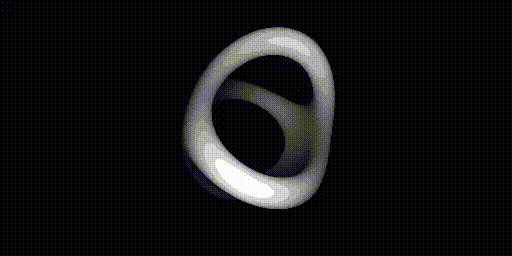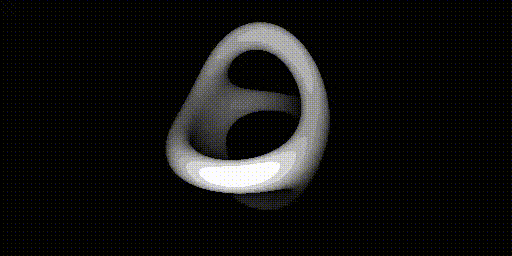
  1. The crazy stuff begins! Beware gyroid
#define GYROID dot(sin(p*9.),cos(p.zxy*9.))*.1
#define SDF(p) GYROID

It is not easy to understand its shape because gyroid is borderless and we are inside https://bit.ly/3070hqe

  1. To understand its shape better we have to intersect it with some other primitive. Why not a sphere?
#define SDF(p) length(vec2(SPHERE, GYROID))-.02

https://bit.ly/30a2scU

You can learn more about gyroids on https://en.wikipedia.org/wiki/Gyroid

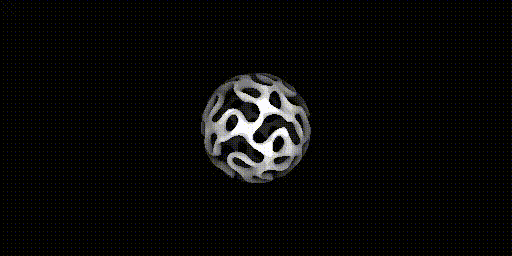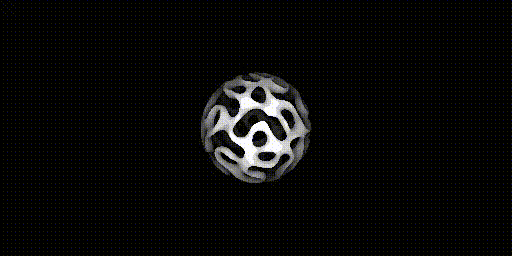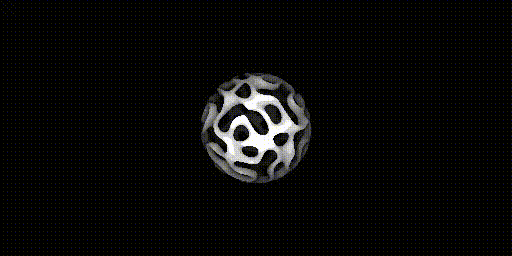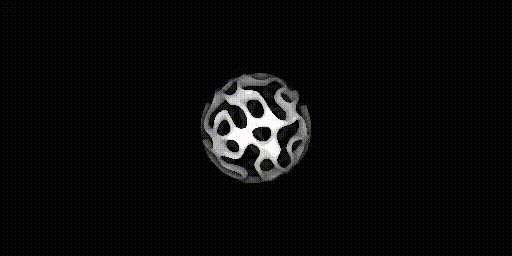
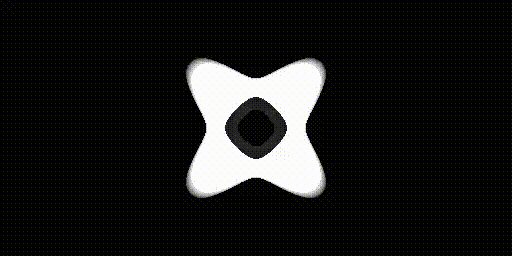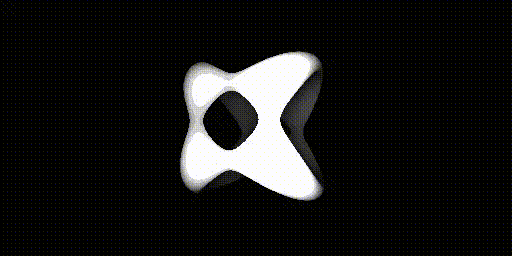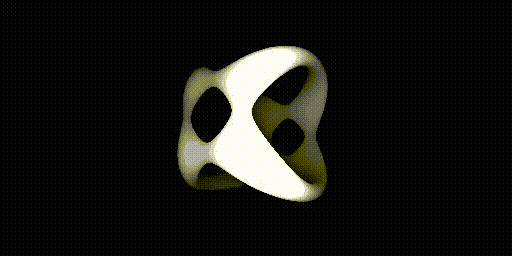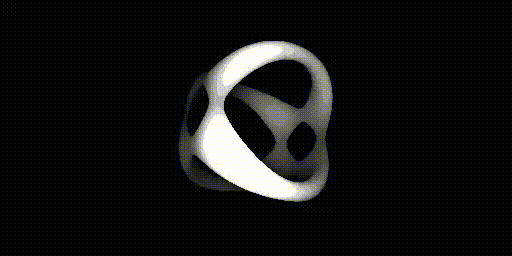
  1. You want to intersect intersections? No problem! We can use our weird shape from twit #7 for that:
#define CROSS_RINGS length(vec2(CYLINDER_Y, CYLINDER_X))-.2
#define SDF(p) length(vec2(CROSS_RINGS, GYROID))-.01

https://bit.ly/3kGYWjr

  1. You can intersect more than two surfaces at once! Use vec3 or vec4 for that:
#define SDF(p) length(vec3(CYLINDER_Y, CYLINDER_X, SPHERE))-.1

https://bit.ly/3uT3ZSu

  1. Aaaaannndddd… the regular sphere SDF is a shortcut of the very same formula!
#define SDF(p) length(vec3(PLANE_X, PLANE_Y, PLANE_Z))-.9

↓

#define SDF(p) length(http://p.xyz)-.9

↓

#define SDF(p) length(p)-.9

I was shocked to learn about it! 🤯

  1. That's it! Try playing with the code, interesting patterns can suddenly appear https://bit.ly/3uSmvdO

It's my first tutorial thread. I would be grateful if you could share your thoughts on the subject, ask questions or brag about the SDFs you discover.